The weak field approximation

We have to check that the appropriate limit, General Relativity leads to Newton's theory. The limit we shall use will be that of small velocities and that time derivatives are much smaller than spatial derivatives.

There are two things we must do:

• We have to relate the geodesic equation to Newton's law of motion.

and

• Relate Einstein's field equations to the Newton- Poisson equation.

Let's assume that we can find a coordinate system which is locally Minkowski [ as demanded by the Equivalence Principle ] and that deviations from flat spacetime are small. This means we can write

where is small. Since we require that , the inverse metric is given by

To work out the geodesic equations we need to work out what the components of the Christoffel symbols are:

Substituting for etc. in terms of we obtain

The geodesic equations are

But for a slowly moving particle so

Also , so we can neglect terms like . The geodesic equation reduces to

so the ``space'' equation (three- acceleration) is

Since we get

Now

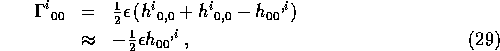
where we have neglected time derivatives over space derivatives. The spatial geodesic equation then becomes

But Newtonian theory has

where is the gravitational potential. So we make the identification

This is equivalent to having spacetime with the line element

This is what we deduced using the Equivalence Principle.

Let's now look at the field equations [ with ]:

Taking the trace we get

This allows us to write the field equations as

Let us assume that the matter takes the form of a perfect fluid, so the stress- energy tensor takes the form:

Taking the trace gives

so the field equations become

The Newtonian limit is . This gives

Look at the 00 component of these equations:

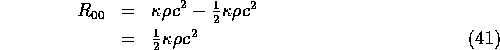
to first order in . Now

to first order in . The (0,0) component of this equation is

and since spatial derivatives dominate over time derivatives, we get

So the field equations are

This is just

Comparing this with Poisson's equation:

we see that we get the same result if the constant is

We can now use this result to write down the full Einstein field equations: